Set Rewrite Rules
From Event-B
| Name | Rule | Side Condition | A/M | |
|---|---|---|---|---|
| * | SIMP_SPECIAL_AND_BTRUE | 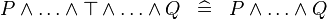 |
A | |
| * | SIMP_SPECIAL_AND_BFALSE |  |
A | |
| * | SIMP_MULTI_AND |  |
A | |
| * | SIMP_MULTI_AND_NOT | 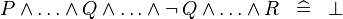 |
A | |
| * | SIMP_SPECIAL_OR_BTRUE |  |
A | |
| * | SIMP_SPECIAL_OR_BFALSE | 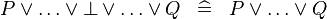 |
A | |
| * | SIMP_MULTI_OR |  |
A | |
| * | SIMP_MULTI_OR_NOT | 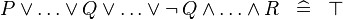 |
A | |
| * | SIMP_SPECIAL_IMP_BTRUE_R | 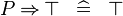 |
A | |
| * | SIMP_SPECIAL_IMP_BTRUE_L | 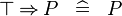 |
A | |
| * | SIMP_SPECIAL_IMP_BFALSE_R | 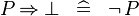 |
A | |
| * | SIMP_SPECIAL_IMP_BFALSE_L | 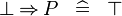 |
A | |
| * | SIMP_MULTI_IMP | 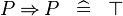 |
A | |
| * | SIMP_MULTI_IMP_OR | 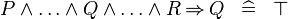 |
A | |
| * | SIMP_MULTI_IMP_AND_NOT_R | 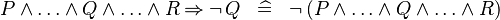 |
A | |
| * | SIMP_MULTI_IMP_AND_NOT_L |  |
A | |
| * | SIMP_MULTI_EQV | 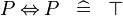 |
A | |
| * | SIMP_MULTI_EQV_NOT |  |
A | |
| * | SIMP_MULTI_EQV_NOT_NOT | 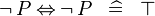 |
A | |
| * | SIMP_SPECIAL_NOT_BTRUE |  |
A | |
| * | SIMP_SPECIAL_NOT_BFALSE | 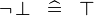 |
A | |
| * | SIMP_NOT_NOT |  |
A | |
| * | SIMP_NOTEQUAL |  |
A | |
| * | SIMP_NOTIN | 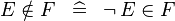 |
A | |
| * | SIMP_NOTSUBSET | 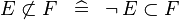 |
A | |
| * | SIMP_NOTSUBSETEQ | 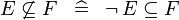 |
A | |
| * | SIMP_NOT_LE | 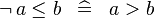 |
A | |
| * | SIMP_NOT_GE | 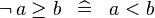 |
A | |
| * | SIMP_NOT_LT | 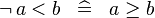 |
A | |
| * | SIMP_NOT_GT |  |
A | |
| * | SIMP_SPECIAL_NOT_EQUAL_FALSE_R | 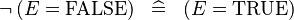 |
A | |
| * | SIMP_SPECIAL_NOT_EQUAL_FALSE_L | 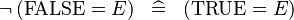 |
A | |
| * | SIMP_SPECIAL_NOT_EQUAL_TRUE_R | 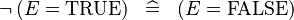 |
A | |
| * | SIMP_SPECIAL_NOT_EQUAL_TRUE_L | 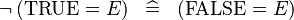 |
A | |
| * | SIMP_FORALL_AND |  |
A | |
| * | SIMP_EXISTS_OR | 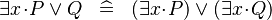 |
A | |
| * | SIMP_FORALL | 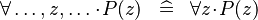 |
A | |
| * | SIMP_EXISTS |  |
A | |
| * | SIMP_MULTI_EQUAL | 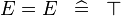 |
A | |
| * | SIMP_MULTI_NOTEQUAL | 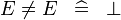 |
A | |
| * | SIMP_EQUAL_MAPSTO | 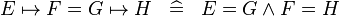 |
A | |
| * | SIMP_EQUAL_SING | 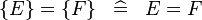 |
A | |
| * | SIMP_SPECIAL_EQUAL_TRUE | 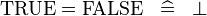 |
A | |
| * | SIMP_TYPE_SUBSETEQ | 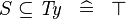 |
where 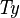 is a type expression is a type expression |
A |
| * | SIMP_SUBSETEQ_SING | 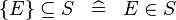 |
where 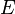 is a single expression is a single expression |
A |
| * | SIMP_SPECIAL_SUBSETEQ | 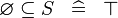 |
A | |
| * | SIMP_MULTI_SUBSETEQ | 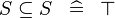 |
A | |
| * | SIMP_SUBSETEQ_BUNION | 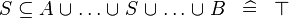 |
A | |
| * | SIMP_SUBSETEQ_BINTER | 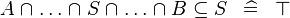 |
A | |
| * | DERIV_SUBSETEQ_BUNION | 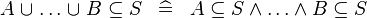 |
M | |
| * | DERIV_SUBSETEQ_BINTER | 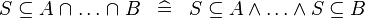 |
M | |
| * | SIMP_SUBSET_BUNION |  |
A | |
| * | SIMP_SUBSET_BINTER | 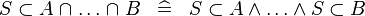 |
A | |
| * | SIMP_SPECIAL_IN | 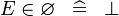 |
A | |
| * | SIMP_MULTI_IN | 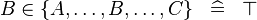 |
A | |
| * | SIMP_IN_SING | 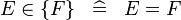 |
A | |
| * | SIMP_MULTI_SETENUM | 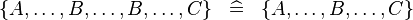 |
A | |
| * | SIMP_SPECIAL_BINTER | 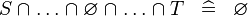 |
A | |
| * | SIMP_TYPE_BINTER | 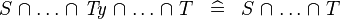 |
where 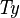 is a type expression is a type expression |
A |
| * | SIMP_MULTI_BINTER | 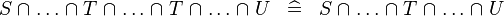 |
A | |
| * | SIMP_MULTI_EQUAL_BINTER |  |
A | |
| * | SIMP_SPECIAL_BUNION |  |
A | |
| * | SIMP_TYPE_BUNION | 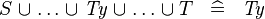 |
where 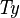 is a type expression is a type expression |
A |
| * | SIMP_MULTI_BUNION | 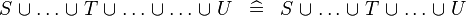 |
A | |
| * | SIMP_MULTI_EQUAL_BUNION |  |
A | |
| * | SIMP_MULTI_SETMINUS | 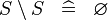 |
A | |
| * | SIMP_SPECIAL_SETMINUS_R | 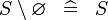 |
A | |
| * | SIMP_SPECIAL_SETMINUS_L | 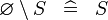 |
A | |
| * | SIMP_TYPE_SETMINUS | 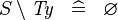 |
where 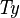 is a type expression is a type expression |
A |
| * | SIMP_TYPE_SETMINUS_SETMINUS | 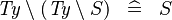 |
where 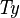 is a type expression is a type expression |
A |
| * | SIMP_TYPE_KUNION | 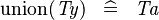 |
where 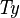 is a type expression and is a type expression and 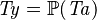 |
A |
| * | SIMP_KUNION_POW | 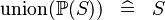 |
A | |
| * | SIMP_KUNION_POW1 | 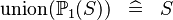 |
A | |
| SIMP_SPECIAL_KUNION | 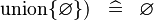 |
A | ||
| SIMP_SPECIAL_QUNION |  |
A | ||
| SIMP_SPECIAL_KINTER | 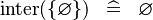 |
A | ||
| * | SIMP_TYPE_KINTER | 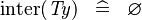 |
where 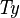 is a type expression is a type expression |
A |
| SIMP_SPECIAL_POW | 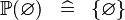 |
A | ||
| SIMP_SPECIAL_POW1 | 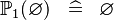 |
A | ||
| SIMP_SPECIAL_CPROD_R | 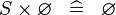 |
A | ||
| SIMP_SPECIAL_CPROD_L |  |
A | ||
| * | SIMP_COMPSET_EQUAL | 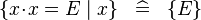 |
where 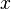 non free in non free in 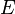 |
A |
| * | SIMP_COMPSET_IN |  |
where 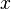 non free in non free in  |
A |
| SIMP_SPECIAL_COMPSET_BFALSE | 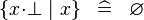 |
A | ||
| SIMP_SPECIAL_COMPSET_BTRUE |  |
where the type od 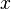 is is 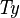 |
A | |
| * | SIMP_SUBSETEQ_COMPSET_L | 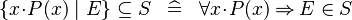 |
where 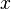 non free in non free in  |
A |
| SIMP_SPECIAL_EQUAL_COMPSET | 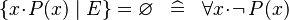 |
A | ||
| * | SIMP_IN_COMPSET | 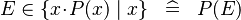 |
A | |
| * | SIMP_SUBSETEQ_COMPSET_R | 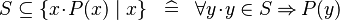 |
where  non free in non free in  |
A |
| SIMP_SPECIAL_OVERL | 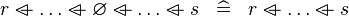 |
A | ||
| * | SIMP_MULTI_OVERL | 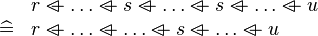 |
A | |
| * | SIMP_TYPE_OVERL_CPROD | 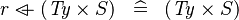 |
where 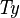 is a type expression is a type expression |
A |
| * | SIMP_SPECIAL_KBOOL_BTRUE | 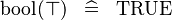 |
A | |
| * | SIMP_SPECIAL_KBOOL_BFALSE | 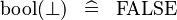 |
A | |
| * | DISTRI_SUBSETEQ_BUNION_SING | 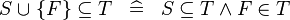 |
where  is a single expression is a single expression |
M |
| DEF_FINITE | 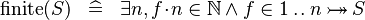 |
M | ||
| * | SIMP_SPECIAL_FINITE | 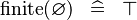 |
A | |
| * | SIMP_FINITE_SETENUM | 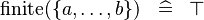 |
A | |
| * | SIMP_FINITE_BUNION | 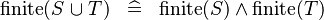 |
A | |
| * | SIMP_FINITE_POW |  |
A | |
| * | DERIV_FINITE_CPROD |  |
A | |
| * | SIMP_FINITE_CONVERSE |  |
A | |
| * | SIMP_FINITE_UPTO | 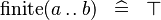 |
A | |
| * | SIMP_FINITE_BINTER_L | 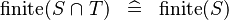 |
M | |
| * | SIMP_FINITE_BINTER_R | 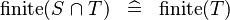 |
M | |
| * | SIMP_FINITE_SETMINUS |  |
M | |
| * | SIMP_FINITE_DOMRES |  |
M | |
| * | SIMP_FINITE_RANRES | 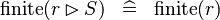 |
M | |
| * | SIMP_FINITE_DOMSUB | 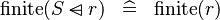 |
M | |
| * | SIMP_FINITE_RANSUB | 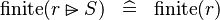 |
M | |
| * | SIMP_FINITE_RELIMAGE | ![\finite (r[S]) \;\;\defi\;\; \finite (r)](/images/math/5/c/e/5ce3a57b43258dc4a25597542670990a.png) |
M | |
| * | SIMP_FINITE_CPROD | 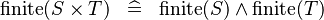 |
M | |
| * | SIMP_FINITE_OVERL | 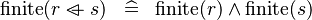 |
M | |
| * | SIMP_FINITE_REL |  |
M | |
| * | SIMP_FINITE_FCOMP | 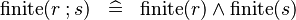 |
M | |
| * | SIMP_FINITE_BCOMP | 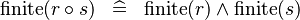 |
M | |
| * | SIMP_FINITE_DPROD | 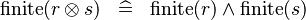 |
M | |
| * | SIMP_FINITE_PPROD | 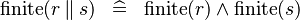 |
M | |
| * | SIMP_FINITE_COMPSET | 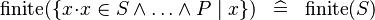 |
where 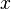 non free in non free in  |
M |
| * | SIMP_FINITE_RAN | 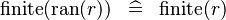 |
M | |
| * | SIMP_FINITE_DOM | 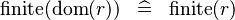 |
M | |
| * | SIMP_FINITE_QUNION | 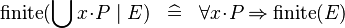 |
M | |
| * | SIMP_FINITE_QINTER | 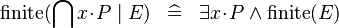 |
M | |
| * | SIMP_FINITE_ID | 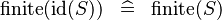 |
A | |
| * | SIMP_FINITE_NATURAL | 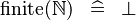 |
A | |
| * | SIMP_FINITE_NATURAL1 | 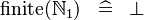 |
A | |
| * | SIMP_FINITE_INTEGER | 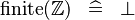 |
A | |
| * | SIMP_FINITE_LAMBDA | 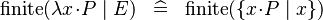 |
A | |
| * | SIMP_TYPE_EQUAL_EMPTY | 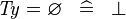 |
where 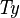 is a type expression is a type expression |
A |
| * | SIMP_TYPE_IN | 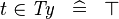 |
where 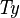 is a type expression is a type expression |
A |
| SIMP_SPECIAL_FORALL_BTRUE |  |
A | ||
| SIMP_SPECIAL_FORALL_BFALSE |  |
A | ||
| SIMP_SPECIAL_EXISTS_BTRUE |  |
A | ||
| SIMP_SPECIAL_EXISTS_BFALSE |  |
A | ||
| * | SIMP_SPECIAL_EQV_BTRUE |  |
A | |
| * | SIMP_SPECIAL_EQV_BFALSE | 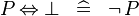 |
A | |
| SIMP_SPECIAL_SUBSET_R |  |
A | ||
| SIMP_SPECIAL_SUBSET_L | 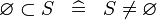 |
A | ||
| * | SIMP_TYPE_SUBSET_L | 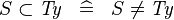 |
where 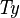 is a type expression is a type expression |
A |
| * | SIMP_MULTI_SUBSET | 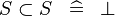 |
A | |
| * | DISTRI_AND_OR |  |
M | |
| * | DISTRI_OR_AND | 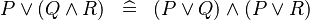 |
M | |
| * | DEF_OR |  |
M | |
| * | DERIV_IMP | 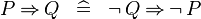 |
M | |
| * | DERIV_IMP_IMP | 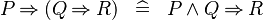 |
M | |
| * | DISTRI_IMP_AND | 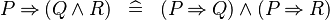 |
M | |
| * | DISTRI_IMP_OR | 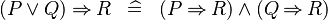 |
M | |
| * | DEF_EQV | 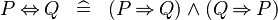 |
M | |
| * | DISTRI_NOT_AND |  |
M | |
| * | DISTRI_NOT_OR |  |
M | |
| * | DERIV_NOT_IMP |  |
M | |
| * | DERIV_NOT_FORALL | 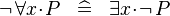 |
M | |
| * | DERIV_NOT_EXISTS |  |
M | |
| * | DEF_SPECIAL_NOT_EQUAL | 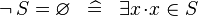 |
M | |
| * | DEF_IN_MAPSTO | 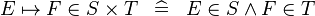 |
M | |
| * | DEF_IN_POW |  |
M | |
| * | DEF_SUBSETEQ | 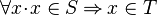 |
M | |
| * | DEF_IN_BUNION | 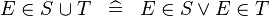 |
M | |
| * | DEF_IN_BINTER | 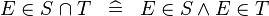 |
M | |
| * | DEF_IN_SETMINUS | 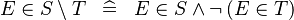 |
M | |
| * | DEF_IN_SETENUM | 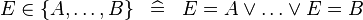 |
M | |
| * | DEF_IN_KUNION |  |
M | |
| * | DEF_IN_QUNION | 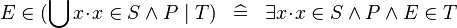 |
M | |
| * | DEF_IN_KINTER | 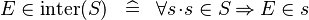 |
M | |
| * | DEF_IN_QINTER | 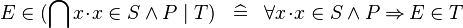 |
M | |
| * | DISTRI_BUNION_BINTER | 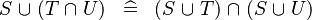 |
M | |
| * | DISTRI_BINTER_BUNION | 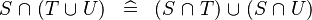 |
M | |
| * | DISTRI_BINTER_SETMINUS | 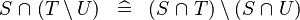 |
M | |
| * | DISTRI_SETMINUS_BUNION |  |
M | |
| * | DERIV_TYPE_SETMINUS_BINTER | 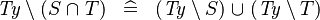 |
where 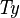 is a type expression is a type expression |
M |
| * | DERIV_TYPE_SETMINUS_BUNION |  |
where 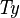 is a type expression is a type expression |
M |
| * | DERIV_TYPE_SETMINUS_SETMINUS | 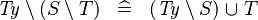 |
where 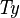 is a type expression is a type expression |
M |
| * | DISTRI_CPROD_BINTER | 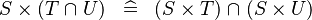 |
M | |
| * | DISTRI_CPROD_BUNION | 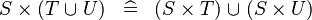 |
M | |
| * | DISTRI_CPROD_SETMINUS | 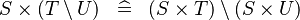 |
M | |
| * | DERIV_SUBSETEQ | 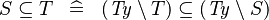 |
where 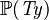 is the type of is the type of  and and 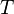 |
M |
| * | DERIV_EQUAL | 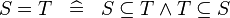 |
where 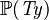 is the type of is the type of  and and 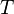 |
M |
| * | DERIV_SUBSETEQ_SETMINUS_L | 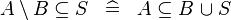 |
M | |
| * | DERIV_SUBSETEQ_SETMINUS_R | 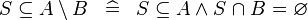 |
M | |
| * | DEF_PARTITION | 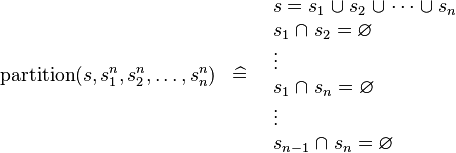 |
AM |